This utility lets you draw colorful and custom Sierpinski fractals. You can adjust the parameters of the initial triangle, such as its color and size, and generate as many fractal iterations from it as you want. The size can be specified by adjusting the width and height of the space where the fractal is constructed, as well as adjusting padding from the edges of the drawing area. You can also choose the direction which the vertex of the triangle will be pointing and specify the thickness of triangles' border. There are three colors you can adjust – color for the drawing space, triangles' border, and internal fill of triangles. Fun fact - if you write the binomial coefficients in a pyramid shape (Pascal's triangle shape) and color all odd numbers with black color and even numbers with white color, then the Sierpinski triangle will form. Created by fractal fans from team Browserling. Fractabulous!
This utility lets you draw colorful and custom Sierpinski fractals. You can adjust the parameters of the initial triangle, such as its color and size, and generate as many fractal iterations from it as you want. The size can be specified by adjusting the width and height of the space where the fractal is constructed, as well as adjusting padding from the edges of the drawing area. You can also choose the direction which the vertex of the triangle will be pointing and specify the thickness of triangles' border. There are three colors you can adjust – color for the drawing space, triangles' border, and internal fill of triangles. Fun fact - if you write the binomial coefficients in a pyramid shape (Pascal's triangle shape) and color all odd numbers with black color and even numbers with white color, then the Sierpinski triangle will form. Created by fractal fans from team Browserling. Fractabulous!
This online browser-based tool allows you to create your own unique Sierpinski fractals. The Sierpinski fractal is one of the most popular fractals. Because of its triangular form and 3-fold symmetry, it's also known as Sierpinski triangle and it's constructed from the set of triangles. It was first created and researched by the Polish mathematician Wacław Franciszek Sierpinski in 1915, although the triangular patterns it creates had been encountered many centuries before. To get a Sierpinski fractal, you start with a solid triangle and in the first step of construction remove an inverted triangle from its center. You're left with three triangles. In the second step, you now remove three inverted triangles from the remaining three triangles. This process continues as long as necessary. The number of filled triangles at the nth iteration step is equal to 3ⁿ⁻¹, and their size is s×4¹⁻ⁿ, where s is the size of the initial triangle. The limiting shape has zero area but the sum of all triangle edges has infinite length. Mind blowing and ingenious at the same time, or as we love to say – fractabulous!
This online browser-based tool allows you to create your own unique Sierpinski fractals. The Sierpinski fractal is one of the most popular fractals. Because of its triangular form and 3-fold symmetry, it's also known as Sierpinski triangle and it's constructed from the set of triangles. It was first created and researched by the Polish mathematician Wacław Franciszek Sierpinski in 1915, although the triangular patterns it creates had been encountered many centuries before. To get a Sierpinski fractal, you start with a solid triangle and in the first step of construction remove an inverted triangle from its center. You're left with three triangles. In the second step, you now remove three inverted triangles from the remaining three triangles. This process continues as long as necessary. The number of filled triangles at the nth iteration step is equal to 3ⁿ⁻¹, and their size is s×4¹⁻ⁿ, where s is the size of the initial triangle. The limiting shape has zero area but the sum of all triangle edges has infinite length. Mind blowing and ingenious at the same time, or as we love to say – fractabulous!
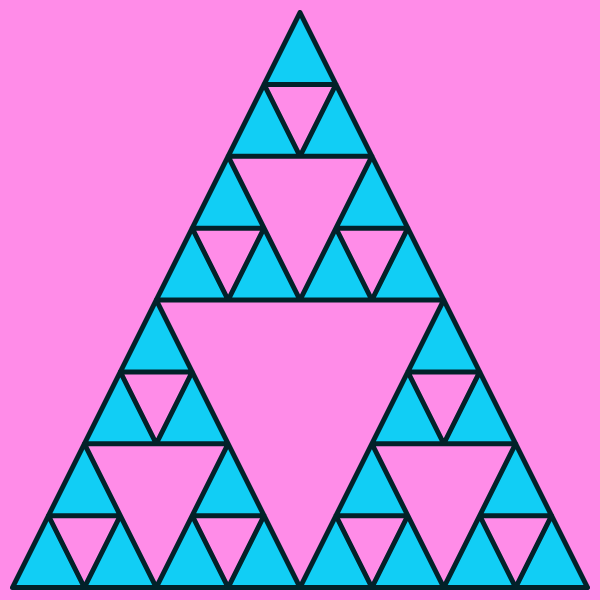
In this example, we generate a multi-colored Sierpinski fractal at its 4th iteration stage, which means that it's built from 3⁴⁻¹ = 3³ = 27 triangles. We draw the triangles in Daintree color, fill them with a bright turquoise color and use lavender-rose color for the canvas area of a size 600 by 600 pixels. We also set 10-pixel padding for the triangle.
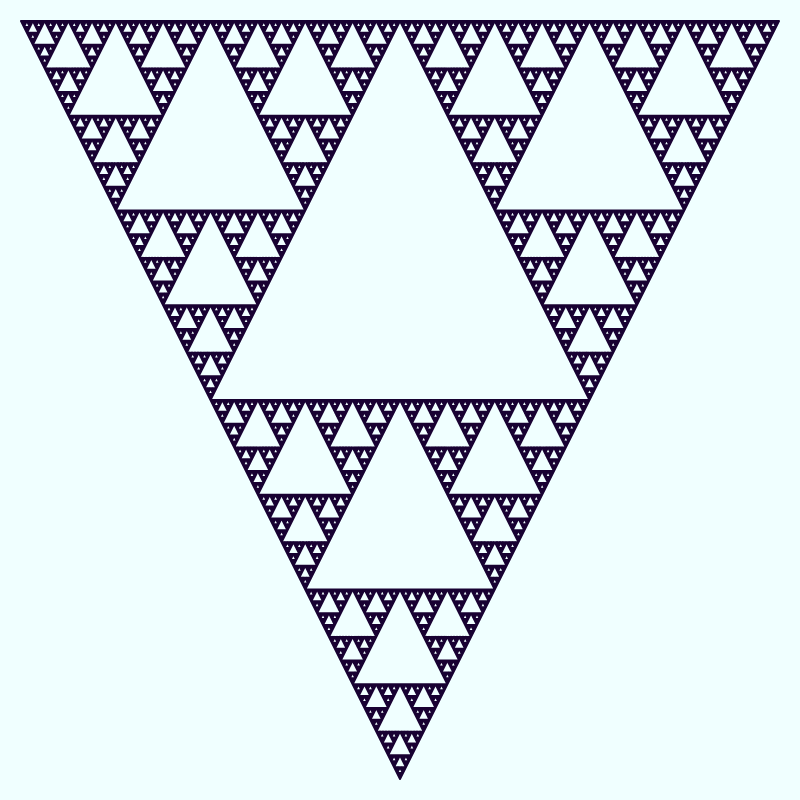
This example uses a 2-pixel line to draw an upside-down Sierpinski triangle of depth 10. The tiny triangles in the bigger triangle aren't filled with any color and are transparent. Only their border in a tolopea color is drawn on a twilight blue canvas.
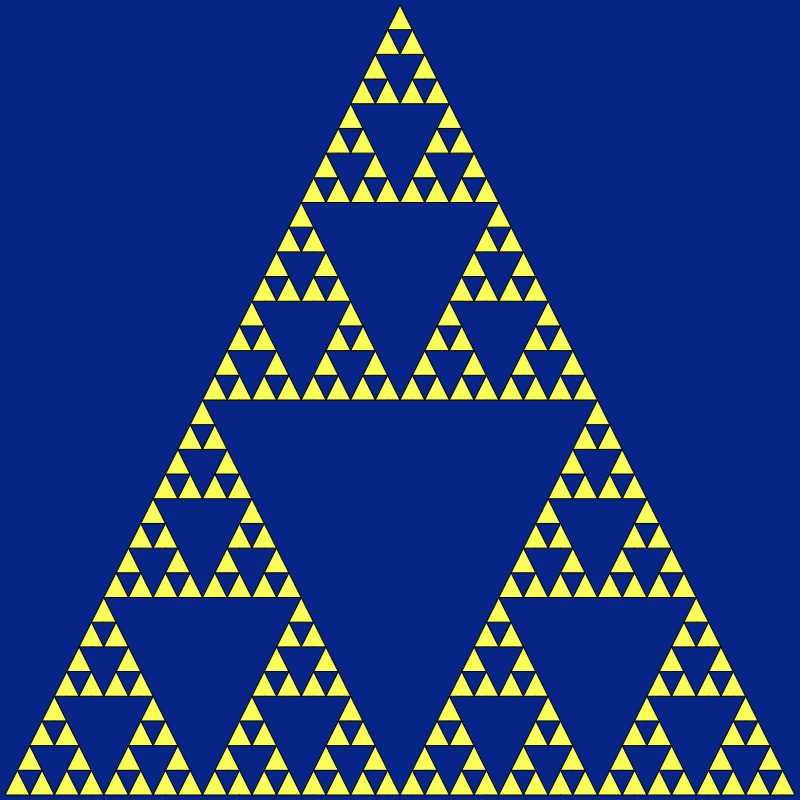
In this example, we form a Sierpinski fractal from filled triangles that are all connected by their vertices. We've left the border color empty so there's no color used for drawing the edges of triangles. We illustrate the sixth recursion step and there are 3⁶⁻¹ = 3⁵ = 243 triangles, each 1024 times smaller than the original triangle.
You can pass options to this tool using their codes as query arguments and it will automatically compute output. To get the code of an option, just hover over its icon. Here's how to type it in your browser's address bar. Click to try!
Walk the Hilbert fractal and enumerate its coordinates.
Walk the Peano fractal and enumerate its coordinates.
Walk the Moore fractal and enumerate its coordinates.
Encode the Hilbert fractal as a string.
Encode the Peano fractal as a string.
Encode the Moore fractal as a string.
Encode the Cantor set as a string.
Encode the Heighway Dragon as a string.
Encode the Sierpinski fractal as a string.
Generate a Sierpinski tetrahedron (tetrix) fractal.
Generate a Cantor's cube fractal.
Generate a Sierpinski-Menger fractal.
Generate a Jerusalem cube fractal.
Generate a Jeaninne Mosely fractal.
Generate a Mandelbrot tree fractal.
Generate a Barnsley's tree fractal.
Generate a Barnsley's fern fractal.
Generate a binary tree fractal.
Generate a ternary tree fractal.
Generate a dragon tree fractal.
Generate a de Rham curve.
Generate a Takagi-Landsberg fractal curve.
Generate a Peano pentagon fractal curve.
Generate a tridendrite fractal curve.
Generate a Pentigree fractal curve.
Generate a lucky seven fractal curve.
Generate an Eisenstein fractions fractal curve.
Generate a Bagula double five fractal curve.
Generate a Julia fractal set.
Generate a Mandelbrot fractal set.
Generate a Mandelbulb fractal.
Generate a Mandelbox fractal.
Generate a Buddhabrot fractal.
Generate a Burning Ship fractal.
Generate a toothpick sequence fractal.
Generate an Ulam-Warburton fractal curve.
Generate an ASCII fractal.
Generate an ANSI fractal.
Generate a Unicode fractal.
Generate an emoji fractal.
Generate a braille code fractal.
Generate a fractal in audio form.
Create a fractal that looks like one but isn't a fractal.
Generate a fractal from any text.
Generate a fractal from a string.
Generate a fractal from a number.
Join any two fractals together.
Create a completely random fractal.
Set up an arbitrary IFS system and iterate it.
Recursively transform an image using IFS rules.
Run infinite compositions of analytic functions.
Create a surface that mimics a natural terrain.
Create a fractal surface via Brownian motion.
Apply fractal algorithms on your image and make it self-similar.
Find fractal patterns in any given image.
Find fractal patterns in any given text.
Find fractal patterns in any given number.
Tessellate a plane with fractals.
Run a cellular automaton with custom rules.
Play Conway's Game of Life on an infinite grid.
Subscribe to our updates. We'll let you know when we release new tools, features, and organize online workshops.
Enter your email here
We're Browserling — a friendly and fun cross-browser testing company powered by alien technology. At Browserling our mission is to make people's lives easier, so we created this collection of fractal tools. Our tools have the simplest user interface that doesn't require advanced computer skills and they are used by millions of people every month. Our fractal tools are actually powered by our web developer tools that we created over the last couple of years. Check them out!